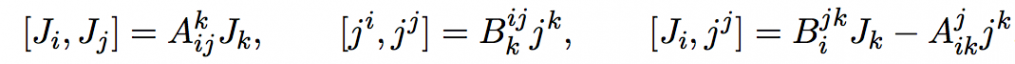In this paper (arXiv:2404.01073), integrable deformations of a class of Rikitake dynamical systems are constructed by deforming their underlying Lie-Poisson Hamiltonian structures as linearizations of Poisson–Lie structures on certain (dual) Lie groups. By taking into account that there exists a one-to one correspondence between Poisson–Lie groups and Lie bialgebra structures, a number of deformed Poisson coalgebras can be obtained, which allow the construction of integrable deformations of coupled Rikitake systems. Moreover, the integrals of the motion for coupled systems can be explicitly obtained by means of the deformed coproduct map. The same procedure can be also applied when the initial system is bi-Hamiltonian with respect to two different Lie-Poisson algebras. In this case, to preserve a bi-Hamiltonian structure under deformation, a common Lie bialgebra structure for the two Lie-Poisson structures has to be found. Coupled dynamical systems arising from this bi-Hamiltonian deformation scheme are also presented, and the use of collective `cluster variables’, turns out to be enlightening in order to analyse their dynamical behaviour. As a general feature, the approach here presented provides a novel connection between Lie bialgebras and integrable dynamical systems.

In this work (arXiv:2403.19520), we present the technical details of the discussion presented in [J.J. Relancio, L.Santamaría-Sanz (2024) arXiv:2403.18772], where we establish the basis of quantum theories of the free massive scalar, the massive fermionic, and the electromagnetic fields, in a doubly special relativity scenario. This construction is based on a geometrical interpretation of the kinematics of these kind of theories. In order to describe the modified actions, we find that a higher (indeed infinite) derivative field theory is needed, from which the deformed kinematics can be read. From our construction we are able to restrict the possible models of doubly special relativity to particular bases that preserve linear Lorentz invariance. We quantize the theories and also obtain a deformed version of the Maxwell equations. We analyze the electromagnetic vector potential either for an electric point-like source and a magnetic dipole. We observe that the electric and magnetic fields do not diverge at the origin for some models described with an anti de Sitter space but do for the de Sitter one in both problems.

Non-local quantum field theories could be a solution to the inconsistencies arising when quantizing gravity. Doubly special relativity is regarded as a low-energy limit of a quantum gravity theory with testable predictions. In this paper (arXiv:2403.18772) we present a new formulation of quantum field theories in doubly special relativity with non-local behavior. Our construction restricts the models to those showing linear Lorentz invariance. The deformed Klein–Gordon, Dirac, and electromagnetic Lagrangians are derived. The deformed Maxwell equations and the electric potential of a point charge are discussed.

In this short review (arXiv:2403.06652) we present the key definitions, ideas and techniques involved in the study of symmetry resolved entanglement measures, with a focus on the symmetry resolved entanglement entropy. In order to be able to define such entanglement measures, it is essential that the theory under study possess an internal symmetry. Then, symmetry resolved entanglement measures quantify the contribution to a particular entanglement measure that can be associated to a chosen symmetry sector. Our review focuses on conformal (gapless/massless/critical) and integrable (gapped/massive) quantum field theories, where the leading computational technique employs symmetry fields known as (composite) branch point twist fields.

In this paper (arXiv:2311.08435) the one-loop quantum corrections to the internal energy of lattices due to the quantum fluctuations of the scalar field of phonons are studied. The band spectrum of the lattice is characterised in terms of the scattering data, allowing to compute the total Helmholtz free energy and the entropy at finite non zero temperature. Some examples of three dimensional periodic potentials built from the repetition of the same punctual or compact supported potential are addressed: the generalised Dirac comb and the Pöschl-Teller comb, respectively.

In this paper (arXiv:2305.01438) the quantum vacuum interaction energy between a pair of semitransparent two-dimensional plates represented by Dirac delta potentials and its first derivative, embedded in the topological background of a sine-Gordon kink is studied through an extension of the TGTG-formula (firstly discovered by O. Kenneth and I. Klich) to weak curved backgrounds. Quantum vacuum oscillations around the sine-Gordon kink solutions are interpreted as a quantum scalar field theory in the spacetime of a domain wall. Moreover, the relation between the phase shift and the density of states (the well-known Dashen-Hasslacher-Neveu formula) is also exploited to characterize the quantum vacuum energy.

In this paper (arXiv:2312.16586) it is shown that the complex Bernoulli differential equations admitting the supplementary structure of a Lie-Hamilton system related to the book algebra can always be solved by quadratures, providing an explicit solution of the equations. In addition, considering the quantum deformation of Bernoulli equations, their canonical form is obtained and an exact solution by quadratures is deduced as well. It is further shown that the approximations of kth-order in the deformation parameter from the quantum deformation are also integrable by quadratures, although an explicit solution cannot be obtained in general. Finally, the multidimensional quantum deformation of the book Lie-Hamilton systems is studied, showing that, in contrast to the multidimensional analogue of the undeformed system, the resulting system is coupled in a non-trivial form.

The unification of quantum mechanics and general relativity has long been elusive. Only recently have empirical predictions of various possible theories of quantum gravity been put to test. The dawn of multi-messenger high-energy astrophysics has been tremendously beneficial, as it allows us to study particles with much higher energies and travelling much longer distances than possible in terrestrial experiments, but more progress is needed on several fronts.
A thorough appraisal of current strategies and experimental frameworks, regarding quantum gravity phenomenology, is provided here (arXiv:2312.00409). Our aim is twofold: a description of tentative multimessenger explorations, plus a focus on future detection experiments.
As the outlook of the network of researchers that formed through the COST Action CA18108 “Quantum gravity phenomenology in the multi-messenger approach (QG-MM)”, in this work we give an overview of the desiderata that future theoretical frameworks, observational facilities, and data-sharing policies should satisfy in order to advance the cause of quantum gravity phenomenology.

This paper (arXiv:2311.16249) introduces and investigates a class of noncommutative spacetimes that I will call “T-Minkowski,” whose quantum Poincaré group of isometries exhibits unique and physically motivated characteristics. Notably, the coordinates on the Lorentz subgroup remain commutative, while the deformation is confined to the translations (hence the T in the name), which act like an integrable set of vector fields on the Lorentz group. This is similar to Majid’s bicrossproduct construction, although my approach allows the description of spacetimes with commutators that include a constant matrix as well as terms that are linear in the coordinates (the resulting structure is that of a centrally-extended Lie algebra). Moreover, I require that one can define a covariant braided tensor product representation of the quantum Poincaré group, describing the algebra of N-points. This also implies that a 4-dimensional bicovariant differential calculus exists on the noncommutative spacetime. The resulting models can all be described in terms of a numerical triangular R-matrix through RTT relations (as well as RXX, RXY and RXdX relations for the homogeneous spacetime, the braiding and the differential calculus). The R-matrices I find are in one-to-one correspondence with the triangular r-matrices on the Poincaré group without quadratic terms in the Lorentz generators. These have been classified, up to automorphisms, by Zakrzewski, and amount to 16 inequivalent models. This paper is the first of two, focusing on the identification of all the quantum Poincaré groups that are allowed by my assumptions, as well as the associated quantum homogeneous spacetimes, differential calculi and braiding constructions.

In this study (arXiv:2310.15063), we construct a 1+1-dimensional, relativistic, free, complex scalar Quantum Field Theory on a noncommutative spacetime characterized by Lie algebra-type commutators, namely, the lightlike version of κ-Minkowski. The associated κ-Poincaré quantum group of isometries is quasitriangular, and its quantum R matrix facilitates the definition of a braided algebra of N points that retains Poincaré invariance. Leveraging our recent findings, we can now represent the generators of the deformed oscillator algebra as nonlinear redefinitions of undeformed oscillators, which are nonlocal in momentum space. In this representation, the momentum, boost, and charge conjugation operators remain undeformed. The deformation is confined to the creation and annihilation operators. However, this deformation only manifests at the multiparticle level, as the one particle (and antiparticle) states are identical to the undeformed ones. We successfully introduce a covariant, involutive deformed flip operator using the R matrix. The corresponding deformed (anti-)symmetrization operators are covariant and idempotent. We conclude by noticing that P and T are not symmetries of the theory, although PT (and hence CPT) is.